The Baer-Specker Group

One of the infinite abelian groups that is important to infinite abelian group theory and which has shown up naturally in previous posts on infinitary fundamental groups is the Baer-Specker group, often just called the Specker group. This post isn’t all that topological, but I think it’s appropriate considering that infinite group theory is becoming increasingly important to this kind of infinite product-driven algebraic topology.

The Baer-Specker group is the countably infinite direct product of copies of the additive group of integers:

$\displaystyle\prod_{n=1}^{\infty}\mathbb{Z}=\mathbb{Z}\times\mathbb{Z}\times...$

The elements* of the Specker group are sequences $(a_n)$ of integers $a_n\in\mathbb{Z}$ and addition is component-wise $(a_n)+(b_n)=(a_n+b_n)$ .

We’ll write $s_n=(0,0,..,0,1,0,0,...)$ for the sequence whose only non-zero component is a $1$ in the n-th position. The countable set $\{s_n|n\geq 1\}$ forms a basis for the free abelian subgroup $\bigoplus_{n=1}^{\infty}\mathbb{Z}$ consisting of the sequences $(a_1,a_2,...,a_n,0,0,...)$ that terminate in $0$ ‘s.

But the Specker group $\prod_{n=1}^{\infty}\mathbb{Z}$ is uncountable (as is any countably infinite product of sets with cardinality $>2$ ), which means the set $\{e_n|n\geq 1\}$ cannot generate the whole group – equivalently $\bigoplus_{n=1}^{\infty}\mathbb{Z}$ is proper.

So is $\displaystyle\prod_{n=1}^{\infty}\mathbb{Z}$ a free abelian group or not?

On the face of it, the answer is not obvious. We answered the same question (with a negative answer) for the earring group $\pi_1(\mathbb{E})$ in a two-part post (Part I and Part II). This question about the Specker group is a bit easier since it is an abelian group with simpler combinatorial structure. In this post, I’ll walk through a nice, direct proof of the following theorem.

Theorem 1: $\displaystyle\prod_{n=1}^{\infty}\mathbb{Z}$ is not a free group.

You’ll notice the argument is similar in spirit to the argument used to show $\pi_1(\mathbb{E})$ is not free: basically, we’ll assume the group is free and exploit the infinite structure to find an integer which has infinitely many divisors – something which obviously can’t happen.

*It is also common to view the Specker group as the group 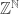$\mathbb{Z}^{\mathbb{N}}$ of functions $f:\mathbb{N}=\{1,2,3,...\}\to\mathbb{Z}$ from the natural numbers to integers.

History

Apparently, Reinhold Baer [1] first proved Theorem 1 in 1937 (or at least stronger results that imply it). Ernst Specker [3] re-proved this result among a number of other original results about $\prod_{n=1}^{\infty}\mathbb{Z}$ . So the foundational theory of $\prod_{n=1}^{\infty}\mathbb{Z}$ is often attributed to Specker as well. The proof I’m using in this post is a nice one from the AMM [2].

Free abelian groups and divisibility

First, let’s review some terminology.

Recall that a basis for an abelian group $A$ is a subset $\{e_{\lambda}\mid\lambda\in\Lambda\}\subset A$ such that every element $a\in A$ can be written uniquely as a linear combination $a=\sum_{\lambda}n_{\lambda}e_{\lambda}$ where $n_{\lambda}=0$ for all but finitely many $\lambda$ . An abelian group $A$ is free if such a basis exists.

We will use the following elementary fact about free abelian groups.

Lemma 2: Suppose $A$ is a free abelian group with basis $\{e_{\lambda}\mid\lambda\in\Lambda\}$ , $J\subset\Lambda$ is a subset, and $H=\langle e_{\lambda}\mid\lambda\in J\rangle$ is the free subgroup generated with basis $\{e_{\lambda}\mid\lambda\in J\}$ . The quotient $A/H$ is a free abelian group for which the set of cosets $\{e_{\lambda}+H \mid\lambda\in\Lambda\backslash J\}$ form a basis.

Also, we’ll deal with some divisibility issues.

If $A$ is an abelian group, $a\in A$ a non-zero element, then we say a natural number $n$ divides $a$ if

$\displaystyle a=nx=\underbrace{x+x+...+x}_\text{n terms}$

for some $x\in A$ . We call $n$ a divisor of $a$ and will be interested in cases when an element has finitely or infinitely many divisors. For example, every integer $a\in \mathbb{Z}$ obviously has only finitely many divisors (using prime decomposition) but every non-zero rational number $\frac{p}{q}\in \mathbb{Q}$ has infinitely many divisors since $\frac{p}{q}=n\left(\frac{p}{nq}\right)$ for every $n\geq 1$ .

Lemma 3: Every non-zero element of a free abelian group has only finitely many divisors.

Proof. Suppose $A$ is a free abelian group with basis $\{e_{\lambda}\mid\lambda\in\Lambda\}$ and $a\in A$ is non-zero. Write $a=\sum_{\lambda}n_{\lambda}e_{\lambda}$ uniquely using the basis. Fix some non-zero coefficient $\lambda_0\in\Lambda$ such that $n_{\lambda_0}\neq 0$ . Notice that if $n$ divides $a$ , then $a=nx_n$ for some $x_n=\sum_{\lambda}m_{\lambda}e_{\lambda}$ . But this means $a$ is equal to both

$nx_n=\sum_{\lambda}(nm_{\lambda})e_{\lambda}$ and $\sum_{\lambda}n_{\lambda}e_{\lambda}$ .

By the uniqueness of the representation of $a$ in the given basis $n_{\lambda_0}$ is equal to $nm_{\lambda}$ for some $\lambda$ .

We have just argued that whenever $n$ divides $a$ , then $n$ also divides the integer $n_{\lambda_0}$ . Since integers only have finitely many divisors, $a$ can only have finitely many divisors. $\square$

The Specker group is not free

Proof of Theorem 1. Suppose $G=\prod_{n=1}^{\infty}\mathbb{Z}$ is a free abelian group with basis $\{e_{\lambda}|\lambda\in\Lambda\}$ . Since $G$ is uncountable, the indexing set $\Lambda$ must also be uncountable.

Write each element $s_n=(0,0,...,0,1,0,0,...)$ as a finite linear combination $s_n=\sum_{\lambda}m_{\lambda,n}e_{\lambda}$ of basis elements. For fixed $n$ , let $B(n)=\{\lambda\in\Lambda\mid m_{\lambda,n}\neq 0\}$ so that $B=\bigcup_{n=1}^{\infty}B(n)$ is the set of indices $\lambda$ which has non-zero coefficient for some element $s_n$ . Notice that $B$ is countable since it is the countable union of finite sets $B(n)$ . This means the free abelian subgroup $H=\langle e_{\lambda}\mid\lambda\in B\rangle$ generated by the basis $\{e_{\lambda}\mid\lambda\in B\}$ is also countable.

Remark: It is worthwhile to point out that we have constructed $H$ to be the smallest free subgroup (generated by our basis) containing all of the sequences $s_n$ .

Here are a few important observations:

$\bigoplus_{n=1}^{\infty}\mathbb{Z}\subseteq H$ since $s_n\in H$ for each $n\geq 1$ .
Since $\Lambda$ is uncountable, the complement $\Lambda\backslash B$ is also uncountable. Therefore the quotient group $G/H$ is uncountable.
By Lemma 2, $G/H$ is a free abelian group with basis $\{e_{\lambda}+H \mid\lambda\in\Lambda\backslash B\}$ .

Definition: Let’s say a sequence $(a_n)\in G$ is multiplicative if for each $n\geq 1$ , each quotient $\displaystyle\frac{a_{n+1}}{a_n}$ of consecutive terms is an integer not equal to $\pm 1$ (automatically this means $a_n\neq 0$ for any $n$ ). Let $M$ be the set of multiplicative sequences.

For example, $(n!)=(1!,2!,3!,4!,...)$ and $(2^n)=(2,4,8,16,...)$ are multiplicative. Let’s quickly convince ourselves that there are uncountably many multiplicative sequences.

Lemma 4: There are uncountably many multiplicative sequences in $G$ .

Proof. Consider the set of sequences $S=\{(b_n)\in G \mid |b_n|>1\text{ for each }n\}$ . Obviously this set is uncountable since, for instance, it contains the uncountable subset $\prod_{n=1}^{\infty}\{2,3\}=\{(b_n)\in G\mid b_n\in\{2,3\}\}$ . Define a function $f:S\to M$ by $f(b_n)=(b_1,b_1b_2,b_1b_2b_3,...,b_1b_2\cdots b_k,...)$ . Clearly $f$ is an injection and since $M$ has an uncountable subset, $M$ must be uncountable. $\square$

Since $M\subseteq G$ is uncountable and $H\leq G$ is countable, there exists some multiplicative sequence $a=(a_n)\in M$ such that $a \notin H$ .

Recall that $\bigoplus_{n=1}^{\infty}\mathbb{Z}\subseteq H$ and so we have

$h_n=\sum_{k=1}^{n}a_ks_k=(a_1,a_2,....,a_n,0,0,...)\in H$ for each $n\geq 1$ .

Since $a=(a_n)$ is multiplicative, the element

$\displaystyle x_n=\left(0,0,...,0,\frac{a_{n+1}}{a_n},\frac{a_{n+2}}{a_n},\frac{a_{n+3}}{a_n},...\right)$ ,

where the first non-zero term is in the n+1-st position, is a well-defined element of the Specker group. Notice that

$a_nx_n=(0,0,...,0,a_{n+1},a_{n+2},...)=a-h_n\in a+H.$

So in the free abelian group $G/H$ , we have $a+H=a_n(x_n+H)$ for all $n\in\mathbb{N}$ showing that $a+H$ has infinitely many divisors $a_1,a_2,a_3,...$ in $G/H$ . But according to Lemma 3, this cannot happen in a free abelian group so we obtain a contradiction.

This concludes the proof that $G=\prod_{n=1}^{\infty}\mathbb{Z}$ is not free. $\square$

How is all this related to the earring group?

In an earlier post, I wrote up a proof of the fact that $\pi_1(\mathbb{E})$ was not a free (non-abelian) group. The argument was similar the one above for the Specker group but was a bit more sophisticated. Actually, we proved a stronger fact:

Let $g_n=[\ell_n]\in\pi_1(\mathbb{E})$ be the homotopy class of the loop $\ell_n$ which traverses the n-th circle of the earring space once in the counterclockwise direction.

Fact: The homomorphism $\eta:Hom(\pi_1(\mathbb{E}),\mathbb{Z})\to\prod_{n=1}^{\infty}\mathbb{Z}$ to the Specker group given by $\eta(f)=(f(g_n))=(f(g_1),f(g_2),f(g_3),...)$ is injective and has image $\bigoplus_{n=1}^{\infty}\mathbb{Z}$ .

Neither identifying the image of $\eta$ nor proving injectivity of $\eta$ is easy business. See this post if you’re looking for the proof.

Viewing $\mathbb{E}$ as the infinite wedge $\bigvee_{n=1}^{\infty}S^1$ of circles as a subspace of the infinite torus $\prod_{n=1}^{\infty}S^1$ , the inclusion map $\bigvee_{n=1}^{\infty}S^1\to\prod_{n=1}^{\infty}S^1$ induces the surjective homomorphism $\epsilon:\pi_1(\mathbb{E})\to\pi_1\left(\prod_{n=1}^{\infty}S^1\right)=\prod_{n=1}^{\infty}\mathbb{Z}$ to the Specker group. Another way to think about this map is that the n-th component of $\epsilon([\alpha])$ is the winding number of $\alpha$ around the n-th circle. For example, $\epsilon(g_n)=s_n$ and $\epsilon([\ell_1\ell_2\ell_3...])=(1,1,1,...)$ .

In a similar way, let’s define a homomorphism

$\zeta:Hom\left(\prod_{n=1}^{\infty}\mathbb{Z},\mathbb{Z}\right)\to\prod_{n=1}^{\infty}\mathbb{Z}$ , by $\zeta(f)=(f(s_n))=(f(s_1),f(s_2),...)$

and prove the analogous theorem.

Theorem 5: The homomorphism $\zeta:Hom\left(\prod_{n=1}^{\infty}\mathbb{Z},\mathbb{Z}\right)\to\prod_{n=1}^{\infty}\mathbb{Z}$ given by $\eta(f)=(f(g_n))=(f(s_1),f(s_2),f(s_3),...)$ is injective and has image $\bigoplus_{n=1}^{\infty}\mathbb{Z}$ . Consequently, $Hom\left(\prod_{n=1}^{\infty}\mathbb{Z},\mathbb{Z}\right)\cong\bigoplus_{n=1}^{\infty}\mathbb{Z}$ is countable.

Proof. Since $\epsilon(g_n)=s_n$ for each $n\geq 1$ , the following diagram commutes:

Since $\epsilon$ is surjective, $Hom(\epsilon,\mathbb{Z})$ is injective. Since both $\eta$ and $Hom(\epsilon,\mathbb{Z})$ are injective, so is $\zeta$ . Also $Im(\zeta)\subseteq Im(\eta)=\bigoplus_{n=1}^{\infty}\mathbb{Z}$ (since the triangle commutes). For each $n\geq 1$ , let $f_n:\prod_{n=1}^{\infty}\mathbb{Z}\to\mathbb{Z}$ , $f_n((a_n))=a_n$ be the projection onto the n-th coordinate. Then $\zeta(f_n)=s_n$ showing that $Im(\zeta)=\bigoplus_{n=1}^{\infty}\mathbb{Z}$ . $\square$

Theorem 5 implies Theorem 1: The fact that $Hom\left(\prod_{n=1}^{\infty}\mathbb{Z},\mathbb{Z}\right)\cong\bigoplus_{n=1}^{\infty}\mathbb{Z}$ is countable gives another proof that the Specker group is not free since if it the Specker group is free with (necessarily uncountable) basis $B$ , then the group

$Hom\left(\prod_{n=1}^{\infty}\mathbb{Z},\mathbb{Z}\right)=Hom\left(\bigoplus_{b\in B}\mathbb{Z},\mathbb{Z}\right)\cong Set(B,\mathbb{Z})$

of all functions $B\to\mathbb{Z}$ is uncountable.

This viewpoint using the earring group should not be considered an easier proof of Theorem 1 since the hard work is still present on the non-abelian side. On the other hand, exploring the relationship between the two “non-freeness” results – namely that the non-abelian version implies the abelian one – is worth looking at.

References.

[1] R. Baer, Abelian groups without elements of finite order, Duke Math. J. 3 (1937) 68-122.

[2] S. Schroer, Baer’s Result: The infinite product of the integers has no basis, The American Mathematical Monthly 115, No. 7 (2008), 660-663.

[3] E. Specker, Additive Gruppen von Folgen ganzer Zahlen, Port. Math. 9 (1949) 131-140.

I found Specker’s paper freely available here but disappointingly, couldn’t get access to Baer’s paper without using my University library.

8 Responses to The Baer-Specker Group

Pingback: Celebrating the Career and Contributions of Katsuya Eda: Master of the Hawaiian Earring | Wild Topology
Pingback: Higher Dimensional Hawaiian Earrings | Wild Topology
Scot Adams says:

May 22, 2020 at 12:45 pm

Nice exposition. One typo in the proof of nonfreeness: You say H has finitely many cosets, but I think you mean finitely many elements.

LikeLike

- Jeremy Brazas says:
  
  May 22, 2020 at 1:31 pm
  
  Right. Thanks!
  
  LikeLike
  
Robert Bruner says:

June 29, 2020 at 8:15 pm

You may enjoy the fact that the Specker group contains a continuum P_a, for real a \in [0,1], of non-isomorphic subgroups. See

MR0645925 Irwin, John ; Snabb, Tom . A new class of subgroups of Πℵ0Z.
Abelian group theory (Oberwolfach, 1981),
pp. 154–160, Lecture Notes in Math., 874, Springer, Berlin-New York, 1981.

LikeLike

- Jeremy Brazas says:
  
  June 30, 2020 at 2:46 am
  
  Thanks for sharing Bob! I do really like the way that family of subgroup is defined.
  
  LikeLike
  
Pingback: Infinite Commutativity: Part I | Wild Topology
Paul Fabel says:

July 4, 2022 at 12:27 am

Today’s number one hit for google search: Baer Specker not free. Grateful for the helpful walk through.

LikeLike